Self-organization
of sound and light in resonators
Self-organization
is the spontaneous emergence of spatial and temporal order from an
initially disordered or uniform state. It is a symmetry-breaking,
universal phenomenon, and it is ubiquitous in almost all branches of
science (physics, chemistry, biology, or even in social sciences). My
research career begun with the study of these phenomena in optical
resonators, such as lasers and optical parametric oscillators. Extended
(rolls, hexagons) and localized (solitons) patterns where described in
these systems, and this was he subject of my PhD thesis [1-3]. Some
years later, I changed to the study of acoustical
problems. Acoustic waves behave, under given conditions,
in a similar way as optical
waves, and one can expect in principle to observe similar things.
However, as difference from optics, pattern formations has not clearly
demonstrated in acoustics up to now. We are considering the evolution
of the ultrasonic field in acoustic resonators under two conditions:
containing viscous (e.g. glycerine) and nonviscous (e.g. water) fluids.
In the first case [4] the field frequency is manteined (there are no
harmonic generation), but it is coupled to a temperature field
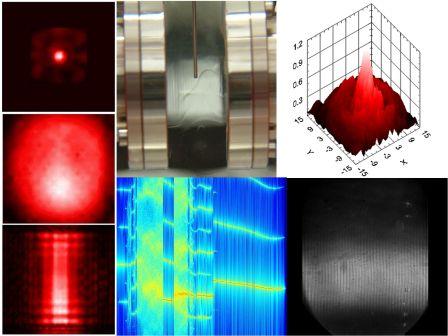
(thermoacoustic interaction). An example of the experimentally obtained
patterns can be observed in the figure. In the second case [5], the
parametric
interaction
of acoustic waves occurs, similar to that obtained in an
optical resonator.
Some
selected publications:
-
G.J.de Valcarcel,
K.Staliunas, V.J.Sanchez-Morcillo, and E.Roldán,Transverse
Patterns in Degenerate Optical Parametric
Oscillation and Degenerate Four-Wave Mixing, Physical Review A
54, 1609-1624 (1996).
-
K.Staliunas
and V.J.Sanchez-Morcillo, Localized
Structures in
Degenerate Optical Parametric Oscillators, Optics Communications
139 306-312 (1997).
-
V.J.
Sánchez-Morcillo, I Pérez-Arjona, F. Silva, G.J.
de Valcárcel and E. Roldán, Vectorial
Kerr-cavity solitons, Optics Letters 25, 957-959 (2000)
-
I.
Pérez-Arjona, Sánchez-Morcillo V.J. y G.J. de
Valcárcel, Ultrasonic
cavity solitons, Europhysics
Letters 82, 100002 (2008)
- Sánchez-Morcillo
V.J., I. Pérez-Arjona y V. Espinosa, Bistable
and dynamic states of parametrically excited ultrasound in a
fluid-filled interferometer,
Journal of the
Acoustical Society of America
(en prensa, 2009)
Wave beams in sonic and photonic crystals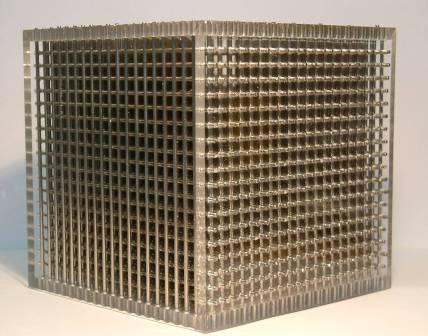
Sonic
and photonic crystals are materials with the acoustical and
optical properties periodically distributed in space. They are now very
famous because their ability to modify the propagation of sound and
light waves, and there are promising applications related with them.
We are interested in the propagation of finite-width beams, and
investigate how the crystals can modifiy the diffraction of the waves.
We have succesfully demonstrated the subdiffractive propagation
(with negligible diffractive spreading) of an acoustic beam, both in
two [1] and
three [2] dimensions (see our 3D crystal in the figure).
Recently, we have also discovered that periodic media have
the ability to act, for particular frequencies, as spatial
filters, improving the beam quality [3]. This effect is specially
important in chirped crystals (whose periodicity slowly changes along
the propagation direction). We also explore the ability of sonic
crystals to focus sound beams, and the design of directional sound
sources based on these materials.
Some selected publications:
1.
Sánchez-Morcillo
V.J., J. Redondo, I. Pérez-Arjona, V. Espinosa y K.
Staliunas, Subdiffractive
propagation in sonic crystals, Physical
Review B 76, 140302(R) (2007)
2. E.
Soliveres, V. Espinosa, I. Pérez-Arjona,
Sánchez-Morcillo V.J. y K. Staliunas, Self
collimation of ultrasound in a 3D sonic
crystal, Applied
Physics Letters 94, 164101 (2009)
3. K.
Staliunas y
Sánchez-Morcillo V.J., Spatial
filtering of light by chirped photonic
crystals, Physical
Review A 79, 053807(2009)
High intensity focused ultrasound
beams
Also known by its achronym HIFU, they are one
of the most extended applications of nonlinear acoustics, mainly in
medicine (lithotripsy, tumoral therapies, etc). However, from the
theoretical viewpoint it is a very complex problem and many fundamental
aspects related with the propagation of intense ultrasound bems are
still not well known. We are investigating how the focusing
characteristics of a beam depend on the systems characteristics,
particularly its aperture (more concretely, its Fresnel number). Low
Fresnel number (low gain) systems show unexpected features, like a
shift of the focal point towards the source, which becomes dynamical in
the nonlinear regime [1,2]. Our study includes also the study of the
transverse amplitude and phase profiles of the radiated ultrasound.
We
have also studied theoretically the possibility of self-trapped
(waveguide) propagation of sound beams
in a
medium with non-inertial (non-thermal) nonlinearity. Although the
existence of these states is well stablished in optics (spatial
solitons), the specific and complicated
features
which are unique to nonlinear acoustics, mainly the absence of
dispersion and higher harmonic generation, makes this problem extremely
challenging [3].
All these studies are done in collaboration with my friend Dr. Yuri N. Makov, from Moscow State University.
Some selected publications:
1. Y.N.
Makov, V.J.
Sánchez-Morcillo, F. Camarena y V. Espinosa, Nonlinear
change of on-axis pressure and intensity
maxima positions and its relation with
the linear focal shift effect,
Ultrasonics
48, 678-685 (2008)
2. Y.N. Makov,
V. Espinosa, V.J. Sánchez-Morcillo, J. Ramis, J.
Cruañes y F. Camarena, Strong
on-axis focal shift
and its nonlinear variation in low-Fresnel number ultrasound beams, Journal
of the Acoustical Society
of America 119,
3618-3626 (2006)
3. Y.
N. Makov and V.J. Sánchez-Morcillo, On
the different waveforms for self-trapped acoustical beams, Acta
Acustica 90, 9-12 (2004)
Waves in nonlinear
lattices and granular media
Lattices are system formed by interacting discrete elements, and appear
naturally in many branches of physics. They describe atomic lattices, macromolecules, spin systems, arrays of optical waveguides, Bose-Einstein
condensates in optical lattices, etc. From the mathematical point of view, these systems can be modeled as periodic arays of nonlinearly coupled oscillators.
I am interested in lattice models representing macroscopic systems,
which can be easily explored experimentally. These include 1D granular
chains, coupled pendula and magnetic lattices. Granular chains consist
in arrays of metalic spheres in contact, loaded by an external force.
When the chain is driven by some external harmonic input, waves
propagate though the chain.
The above studies are
carried out in collaboration Vincent Tournat and Vitalyi Gusev, at the
Université du Maine, Le mans (France)
Physics
of the coastal environments
The beach is a fascinating place for a physicist. Later I will describe here some of my particular interests related to nonlinear dynamical processes in the beach.
What else? So many interesting things to investigate...
